
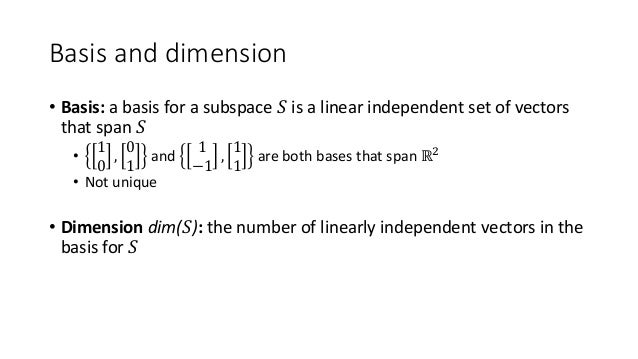
Dimension of a subspace definition series#
We say V -invariant is the graded dimension of an infinite-dimensional graded representation of the monster group, and replacing the dimension with the character gives the McKay–Thompson series for each element of the Monster group. A subset of R n is any collection of points of R n. Any subspace of a finite-dimensional vector space V is itself a finite-dimensional vector space.
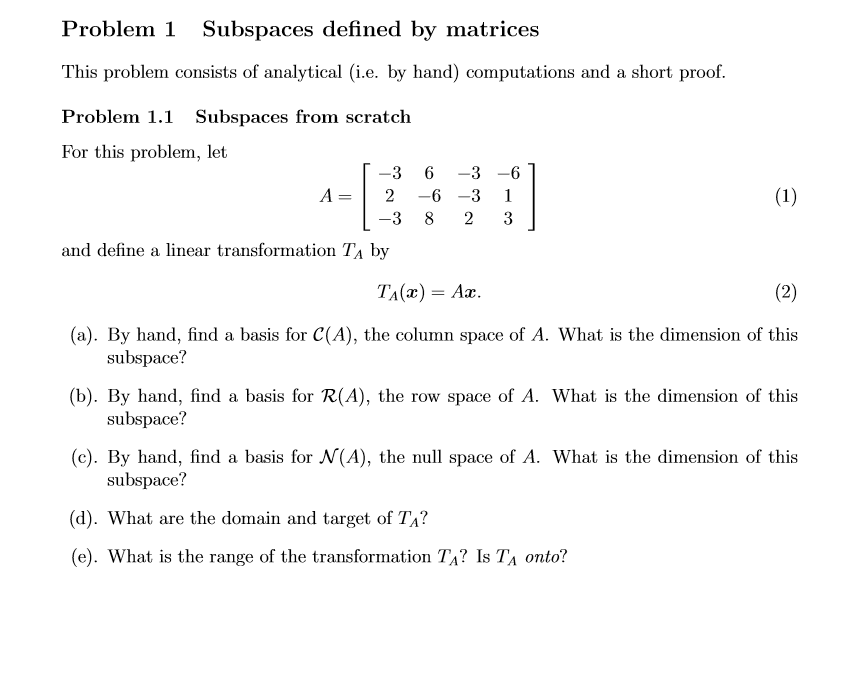
Furthermore, if a basis for H has p elements, then any linearly independent subset of H with p elements is a basis for H, and any subset of H with p entries that spans H is a basis for H. The dimension of a trivial vector space is defined to be zero. It is sometimes called Hamel dimension (after Georg Hamel) or algebraic dimension to distinguish it from other types of dimension.įor every vector space there exists a basis, and all bases of a vector space have equal cardinality as a result, the dimension of a vector space is uniquely defined. Dimension of a Subspace Theorem: (The Basis Theorem) Any two basis for a subspace H of 4 have the same number of elements. the number of vectors) of a basis of V over its base field. Here are what I have gathered so far: The S will be consisting of vectors the like of ( v 1, v 1, v 1, v 4,, v n), ( v 2, v 2, v 2, v 4,, v n) or ( v 3, v 3, v 3, v 4,, v n), etc. The row space and the column space always have the same dimension. Since A is a finite set of linearly independent vectors and spans a subspace, A is a basis for that subspace spanned by A and thus by definition the. The span of the columns of a matrix is called the range or the column space of the matrix. Let V be a finite dimensional vector space of a field F, and. In mathematics, the dimension of a vector space V is the cardinality (i.e. If S is a subspace of R n (here n 3) which consists of vectors V ( v 1, v 2,, v n) with v 1 v 2 v 3, then find the dimension of S. If A is finite set of linearly independent vectors then the dimension of the subspace spanned by A is equal to the number of vectors in A. We define span S as the set of all linear. These vectors form a basis for this subspace.Number of vectors in any basis of the vector space In terms of formulas, it reads as follows: This means in return, that there are no dimensions left for a non-trivial solution. If u is in S and c is a scalar, then cu is in S (that is, S is closed under multiplication by scalars). Ifuandv are in S, thenu+v is in S (that is, S is closed under addition). A subspace of Rn is any collection S of vectors in Rn such that 1. Now, the set M22 of all 2 × 2 real matrices is. MATH10212 Linear Algebra Brief lecture notes 30 Subspaces, Basis, Dimension, and Rank Denition. These are three vectors, which are linearly independent (see post #5), i.e. We have not defined precisely what we mean by bigger or smaller, but intuitively, you know that R3 is bigger.
So in general, given a problem such as this, should I first always try to calculate the general solution to the several equations given, and then make claims about basis and dimension?Īpart from #(1,0,0)#, which you have forgotten, you correctly found #(3,0,2)# and #(−1,2,0)#.


 0 kommentar(er)
0 kommentar(er)
